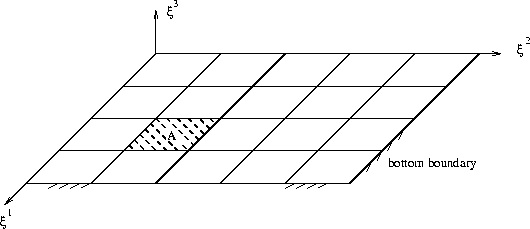
Figure 7.10: There is only one boundary condition type prescribed on A, the ''bottom boundary''-face of a p-cell.
Just as in the 2D case we restrict ourselves to only one boundary, the bottom boundary in the computational domain. First we assume that only one boundary condition type is prescribed on a ''bottom boundary''-face of a p-cell, see Figure 7.10.

Figure 7.10: There is only one boundary condition type prescribed on A, the
''bottom boundary''-face of a p-cell.
It is clear that the only thing that has to be treated very carefully
is the extrapolations of the virtual points, see Figure 7.11.
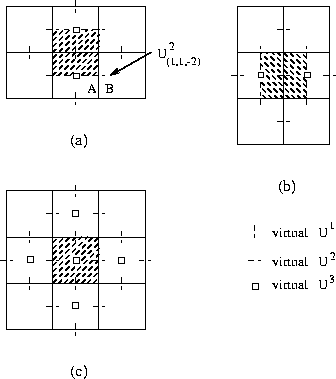
Figure 7.11: A cross-section of the bottom boundary and an (a)
![]() -cell, (b)
-cell, (b) ![]() -cell and (c)
-cell and (c)
![]() -cell, with the positions of the virtual unknowns.
-cell, with the positions of the virtual unknowns.
For the extrapolation of for instance ![]() in a
in a
![]() -cell (see Figure 7.11(a)) we have to consider the boundary
conditions in the two ''bottom boundary''-faces A and B. If boundary
condition type 1 (Dirichlet) or type 3 (semi-natural outflow) is prescribed
in one of the two ''bottom boundary''-faces then the following
extrapolation is used:
-cell (see Figure 7.11(a)) we have to consider the boundary
conditions in the two ''bottom boundary''-faces A and B. If boundary
condition type 1 (Dirichlet) or type 3 (semi-natural outflow) is prescribed
in one of the two ''bottom boundary''-faces then the following
extrapolation is used:
otherwise
It is clear that a similar procedure can be used for all virtual velocities
in the ''tangential'' cells and ''normal'' half cell.