Proof of () and ()

We have to prove (see Figure B.1):

  
Figure: The normal and tangential stress in the physical domain at the boundary .

 

and

 

where

 

 

We start with formula (3.17) from [11]:

 

where is stress tensor in the physical domain.
From and (B.5) we get:

 

so

 

It should be noticed that and are not tensors.
The normal vector pointing in the outside direction of the domain is equal to: if is pointing in the outside direction and otherwise. Formally we can write:

 

From (B.7) and (B.8) we get:

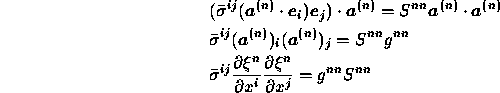
so

Formula (B.2):
The tangential vector is just as in the previous proof equal to:

 

where

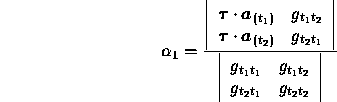
and

From (B.7), (B.8) and (B.9) it follows that:

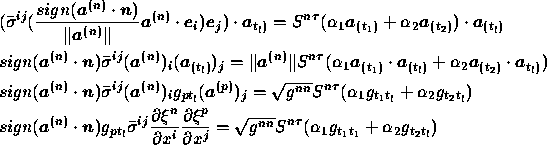
so:

 

where is the stress tensor in the computational domain. For l = 1, 2 we get:

and

or:

so:

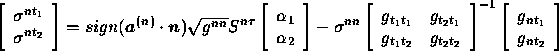
hence:

 

By using Cramers rule in formula (B.11) we get:

 

where

 

and